Plastic number

Triangles with sides in ratio ${\displaystyle \rho }$ form a closed spiral
Rationality	irrational algebraic
Symbol	${\displaystyle \rho }$
Representations
Decimal	1.32471795724474602596...
Algebraic form	real root of ${\displaystyle x^{3}=x+1}$
Continued fraction (linear)	[1;3,12,1,1,3,2,3,2,4,2,141,80,...] [1] not periodic infinite
Binary	1.01010011001000001011...
Hexadecimal	1.5320B74ECA44ADAC1788...

In mathematics, the plastic number is a geometrical proportion close to 53/40. Its true value is the real solution of the equation ${\displaystyle x^{3}=x+1}$.

The adjective plastic does not refer to the artificial material, but to the formative and sculptural qualities of this ratio, as in plastic arts.

Definition

Three quantities a > b > c > 0 are in the plastic ratio if

${\displaystyle {\frac {a}{b}}={\frac {b+c}{a}}={\frac {b}{c}}}$.

The ratio ${\displaystyle {\frac {a}{b}}}$ is commonly denoted ${\displaystyle \rho }$.

Let ${\displaystyle a=\rho \,}$ and ${\displaystyle \,b=1}$, then from ${\displaystyle \,\rho ={\frac {1+c}{\rho }}}$ and ${\displaystyle \,\rho ={\frac {1}{c}}}$

one has ${\displaystyle \rho ^{2}-1=c\,}$ and ${\displaystyle \,c=\rho ^{-1}}$, thus ${\displaystyle \,\rho ^{2}-1=\rho ^{-1}}$.

It follows that the plastic ratio is found as the unique real solution of the cubic equation ${\displaystyle \rho ^{3}-\rho -1=0.}$ The decimal expansion of the root begins as ${\displaystyle 1.324\,717\,957\,244\,746...}$ (sequence A060006 in the OEIS).

Using formulas for the cubic equation, one can show that

${\displaystyle \rho ={\sqrt[{3}]{\frac {9+{\sqrt {69}}}{18}}}+{\sqrt[{3}]{\frac {9-{\sqrt {69}}}{18}}},}$ ^[2]

or, using the hyperbolic cosine,

${\displaystyle \rho ={\frac {2}{\sqrt {3}}}\cosh \left({\frac {1}{3}}\cosh ^{-1}\left({\frac {3{\sqrt {3}}}{2}}\right)\right).}$ ^[3]

${\displaystyle \rho }$ is the superstable fixed point of the iteration ${\displaystyle x\gets (2x^{3}+1)/(3x^{2}-1)}$.

Properties

The plastic ratio ${\displaystyle \rho }$ and golden ratio ${\displaystyle \varphi }$ are the only morphic numbers: real numbers x > 1 for which there exist natural numbers m and n such that

${\displaystyle x+1=x^{m}}$ and ${\displaystyle x-1=x^{-n}}$. ^[4]

Morphic numbers can serve as basis for a system of measure.

Properties of ${\displaystyle \rho }$ (m=3 and n=4) are related to those of ${\displaystyle \varphi }$ (m=2 and n=1). For example, The plastic ratio satisfies the infinitely nested radical

${\displaystyle \rho ={\sqrt[{3}]{1+{\sqrt[{3}]{1+{\sqrt[{3}]{1+\cdots }}}}}}}$,

while for the golden ratio one has

${\displaystyle \varphi ={\sqrt {1+{\sqrt {1+{\sqrt {1+\cdots }}}}}}}$.

The plastic ratio can be expressed in terms of itself as the infinite geometric series

${\displaystyle \rho ^{5}=\sum _{n=0}^{\infty }\rho ^{-n}}$,

in comparison to the golden ratio identity

${\displaystyle \varphi ^{2}=\sum _{n=0}^{\infty }\varphi ^{-n}}$.

Additionally, ${\displaystyle 1+\varphi ^{-1}+\varphi ^{-2}=2}$, while ${\displaystyle \sum _{n=0}^{13}\rho ^{-n}=4.}$

For all powers

${\displaystyle \rho ^{n}=\rho ^{n-2}+\rho ^{n-3}=\rho ^{n-1}+\rho ^{n-5}=\rho ^{n-3}+\rho ^{n-4}+\rho ^{n-5}}$.

Continued fraction pattern of a few low powers

${\displaystyle \rho ^{-1}=[0;1,3,12,1,1,3,2,3,2,...]\approx 0.7549}$ (25/33)

${\displaystyle \ \rho ^{0}=[1]}$

${\displaystyle \ \rho ^{1}=[1;3,12,1,1,3,2,3,2,4,...]\approx 1.3247}$ (45/34)

${\displaystyle \ \rho ^{2}=[1;1,3,12,1,1,3,2,3,2,...]\approx 1.7549}$ (58/33)

${\displaystyle \ \rho ^{3}=[2;3,12,1,1,3,2,3,2,4,...]\approx 2.3247}$ (79/34)

${\displaystyle \ \rho ^{4}=[3;12,1,1,3,2,3,2,4,2,...]\approx 3.0796}$ (40/13)

${\displaystyle \ \rho ^{5}=[4;12,1,1,3,2,3,2,4,2,...]\approx 4.0796}$ (53/13) ...

${\displaystyle \ \rho ^{7}=[7;6,3,1,1,4,1,1,2,1,1,...]\approx 7.1592}$ (93/13) ...

${\displaystyle \ \rho ^{9}=[12;1,1,3,2,3,2,4,2,141,...]\approx 12.5635}$ (88/7)

The plastic ratio is the smallest Pisot number. ^[5] Because the absolute value ${\displaystyle 1/{\sqrt {\rho }}}$ of both algebraic conjugates is smaller than 1, powers of ${\displaystyle \rho }$ generate almost integers. For example: ${\displaystyle \rho ^{29}=3480.00028744...\approx 3480+1/3479}$. After 29 rotation steps the phases of the inward spiraling conjugate pair – initially close to ±45π/58 – nearly align with the imaginary axis.

The minimal polynomial of the plastic ratio ${\displaystyle f(x)=x^{3}-x-1}$ has discriminant ${\displaystyle \Delta =-23}$. Its splitting field over rationals is ${\displaystyle \mathbb {Q} ({\sqrt {\Delta }},\rho )}$. This field is also the Hilbert class field of ${\displaystyle \mathbb {Q} ({\sqrt {\Delta }})}$. Thus, ${\displaystyle \rho }$ can be expressed in terms of the Dedekind eta function ${\displaystyle \eta (\tau )}$ with argument ${\displaystyle \tau =(1+{\sqrt {\Delta }})/2\,}$

${\displaystyle {\frac {e^{\pi i/24}\,\eta (\tau )}{{\sqrt {2}}\,\eta (2\tau )}}=\rho }$. ^[6]

Properties of the related Klein j-invariant ${\displaystyle j(\tau )}$ result in near identity ${\displaystyle e^{\pi {\sqrt {-\Delta }}}\approx \left({\sqrt {2}}\,\rho \right)^{24}-24}$. The difference is < 1/12659.

Van der Laan sequence

In his quest for perceptible clarity, the Dutch Benedictine monk and architect Dom Hans van der Laan (1904-1991) asked for the minimum difference between two sizes, so that we will clearly percieve them as distinct. Also, what is the maximum ratio of two sizes, so that we can still relate them and percieve nearness. According to his observations, the answers are 1/4 and 7/1, spanning a single order of size. ^[7] Requiring proportional continuity, he constructed a geometric series of eight measures (types of size) with common ratio 2 / (3/4 + 1/7^1/7) ≈ ρ. Expressed in countable values, this architectonic system of measure consists of a subset of the numbers that bear his name.

The Van der Laan numbers have a close connection to the Perrin and Padovan sequences. In combinatorics, the number of compositions of n into parts 2 and 3 is counted by the nth Van der Laan number.

The Van der Laan sequence is defined by the third-order recurrence relation

${\displaystyle V_{n}=V_{n-2}+V_{n-3}}$ for n > 2,

with initial values

${\displaystyle V_{1}=0,V_{0}=V_{2}=1}$.

The first few terms are 1, 0, 1, 1, 1, 2, 2, 3, 4, 5, 7, 9, 12, 16, 21, 28, 37, 49, 65, 86,... (sequence A182097 in the OEIS). The limit ratio between consecutive terms is the plastic ratio.

Table of the eight Van der Laan measures

k	n - m	${\displaystyle V_{n}/V_{m}}$	err${\displaystyle (\rho ^{k})}$	interval name
0	3 - 3	1 /1	0	minor element
1	8 - 7	4 /3	1/116	major element
2	10 - 8	7 /4	-1/205	minor piece
3	10 - 7	7 /3	1/116	major piece
4	7 - 3	3 /1	-1/12	minor part
5	8 - 3	4 /1	-1/12	major part
6	13 - 7	16 /3	-1/14	minor whole
7	10 - 3	7 /1	-1/6	major whole

The first 14 indices n for which ${\displaystyle V_{n}}$ is prime are n = 5, 6, 7, 9, 10, 16, 21, 32, 39, 86, 130, 471, 668, 1264 (sequence A112882 in the OEIS). ^[8] The last number has 154 decimal digits.

The generating function of the Van der Laan sequence is given by

${\displaystyle {\frac {1}{1-x^{2}-x^{3}}}=\sum _{n=0}^{\infty }V_{n}x^{n}}$ for ${\displaystyle x<1/\rho }$. ^[9]

The sequence is related to sums of binomial coefficients by

${\displaystyle V_{n}=\sum _{k=\lfloor (n+2)/3\rfloor }^{\lfloor n/2\rfloor }{k \choose n-2k}}$. ^[10]

The characteristic equation of the recurrence is ${\displaystyle x^{3}-x-1=0}$. If the three solutions are real root α and conjugate pair β and γ, the Van der Laan numbers can be computed with the Binet formula ^[10]

${\displaystyle V_{n-3}={\frac {\alpha ^{n}}{2\alpha +3}}+{\frac {\beta ^{n}}{2\beta +3}}+{\frac {\gamma ^{n}}{2\gamma +3}}}$ for n > 2,

Since the magnitude of the two last terms is ${\displaystyle <1/{\sqrt {\alpha ^{n}}}}$ for all n > 4 and ${\displaystyle \alpha =\rho }$, the number ${\displaystyle V_{n}}$ is the nearest integer to ${\displaystyle a\,\rho ^{n}}$, with ${\displaystyle a\approx 0.4115}$.

Geometry

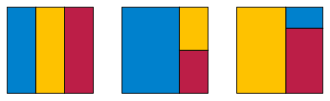
There are precisely three ways of partitioning a square into three similar rectangles: ^{[11] [12]}

1. The trivial solution given by three congruent rectangles with aspect ratio 3:1.
2. The solution in which two of the three rectangles are congruent and the third one has twice the side length of the other two, where the rectangles have aspect ratio 3:2.
3. The solution in which the three rectangles are all of different sizes and where they have aspect ratio ρ². The ratios of the linear sizes of the three rectangles are: ρ (large:medium); ρ² (medium:small); and ρ³ (large:small). The internal, long edge of the largest rectangle (the square's fault line) divides two of the square's four edges into two segments each that stand to one another in the ratio ρ. The internal, coincident short edge of the medium rectangle and long edge of the small rectangle divides one of the square's other, two edges into two segments that stand to one another in the ratio ρ⁴.

The fact that a rectangle of aspect ratio ρ² can be used for dissections of a square into similar rectangles is equivalent to an algebraic property of the number ρ² related to the Routh–Hurwitz theorem: all of its conjugates have positive real part. ^{[13] [14]}

The circumradius of the snub icosidodecadodecahedron for unit edge length is

${\displaystyle {\frac {1}{2}}{\sqrt {\frac {2\rho -1}{\rho -1}}}}$. ^[15]

History and names

${\displaystyle \rho }$ was first studied by Axel Thue in 1912 and by G. H. Hardy in 1919. ^[5] French high school student Gérard Cordonnier discovered the number for himself in 1924 and referred to it as the radiant number ( French: le nombre radiant). Hans van der Laan gave it the name plastic number ( Dutch: het plastische getal) in 1928.

Unlike the names of the golden and silver ratios, the word plastic was not intended by van der Laan to refer to a specific substance, but rather in its adjectival sense, meaning something that can be given a three-dimensional shape. ^[16] This, according to Richard Padovan, is because the characteristic ratios of the number, 3/4 and 1/7, relate to the limits of human perception in relating one physical size to another. Van der Laan designed the 1967 St. Benedictusberg Abbey church to these plastic number proportions. ^[17]

The plastic number is also sometimes called the silver number, a name given to it by Midhat J. Gazalé ^[18] and subsequently used by Martin Gardner, ^[19] but that name is more commonly used for the silver ratio ${\displaystyle 1+{\sqrt {2}},}$ one of the ratios from the family of metallic means first described by Vera W. de Spinadel in 1998. ^[20]

Martin Gardner has suggested referring to ${\displaystyle \rho ^{2}}$ as "high phi", and Donald Knuth created a special typographic mark for this name, a variant of the Greek letter phi ("φ") with its central circle raised, resembling the Georgian letter pari ("Ⴔ"). ^[21]

See also

• Solutions of equations similar to ${\displaystyle x^{3}=x+1}$:
  • Golden ratio – the only positive solution of the equation ${\displaystyle x^{2}=x+1}$
  • Supergolden ratio – the only real solution of the equation ${\displaystyle x^{3}=x^{2}+1}$

Notes

References

External links

• Tales of a Neglected Number by Ian Stewart.
• Plastic rectangle and Padovan sequence at Tartapelago by Giorgio Pietrocola.
• The digital study room of Dom Hans van der Laan at The Van der Laan Archives.
• Harriss, Edmund, "The Plastic Ratio" (video), youtube, Brady Haran, archived from the original on 2021-12-21, retrieved 15 March 2019.